A congruence modulo four in real Schubert calculus

By Nickolas Hein, Frank Sottile, and Igor Zelenko,
    We establish a congruence modulo four in the real Schubert calculus on the Grassmannian of m-planes in 2m-space. This congruence holds for fibers of the Wronski map and a generalization to what we call symmetric Schubert problems. This strengthens the usual congruence modulo two for numbers of real solutions to geometric problems. It also gives examples of geometric problems given by fibers of a map whose degree is zero but where each fiber contains real points.
    We present the resuts of some computational experiments which led us to seek these results.
The manuscript in pdf.
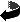
Previous
X (X)6 = 16 on Gr(3;6)
type # real solutions
024 6810 121416
1r 4r1c 21255 35482  17176  7228 18859
1r 2r2c 46357 32374  12268  3654 5347
1r 0r3c 60437 15351  18321  2116 3775
(X)9 = 42 on Gr(3;6)
# real solutions
type 02 468 101214 161820 222426 283032 343638 4042
7r1c  1099  7975 42235  9081  6102 8827  1597  4207 1343  172  17362
5r2c  24495  30089 25992  5054  3632 4114  955  1586 832  63  3188
3r3c  39371  35022  15924  3150  1990  2183  494  622  367  35  842
1r4c     76117  14481  3574  1375  2925  271  364  204  32  477
W W (W)5 = 30 on Gr(4;8)
type # real solutions
02 468 101214 161820 222426 2830
1r0c 1r0c 3r1c     49016 17671  8986  5890 1052  2304  15081
1r0c 1r0c 1r2c  42741  35629 9520  4283  3028 744  828  3227
                      W (W)8 = 90 on Gr(4;8)
type # real solutions
02 468 101214 161820 222426 283032 343638 404244 464850 525456 586062 646668 707274 767880 828486 8890
1r0c 6r1c             1586 510  270  924 118  86  348 44  39  70 123  15  36 16  8  100 1  1  705
1r0c 4r2c     1611 850  423  557 373  195  432 42  61  179 21  23  31 26  5  19 3  1  46       102
1r0c 2r3c  1080  1708 773  394  319 210  85  198 25  23  84 11  9  14 10  2  14    1  15       25
1r0c 0r4c     2756 807  747  162 178  59  95 23  34  89    4  6 5     12       5 1     17
Last modified: Thu Nov 29 21:48:55 CST 2012